current electricity class 12 notes:Current Electricity: Electrical Energy & Power

.ast-archive-entry-banner[data-post-type="post"][data-banner-background-type="custom"]{--wpr-bg-b7da0cda-b4a4-4326-ab57-bc5b9f2cb4d0: url('https://asteriskclasses.com/wp-content/uploads/2024/05/pexels-photo-4144101.jpeg');}

Current electricity class 12 notes:Electrical Energy & Power

Unit 2 • Current Electricity

Understanding energy conversion and power dissipation in electrical circuits

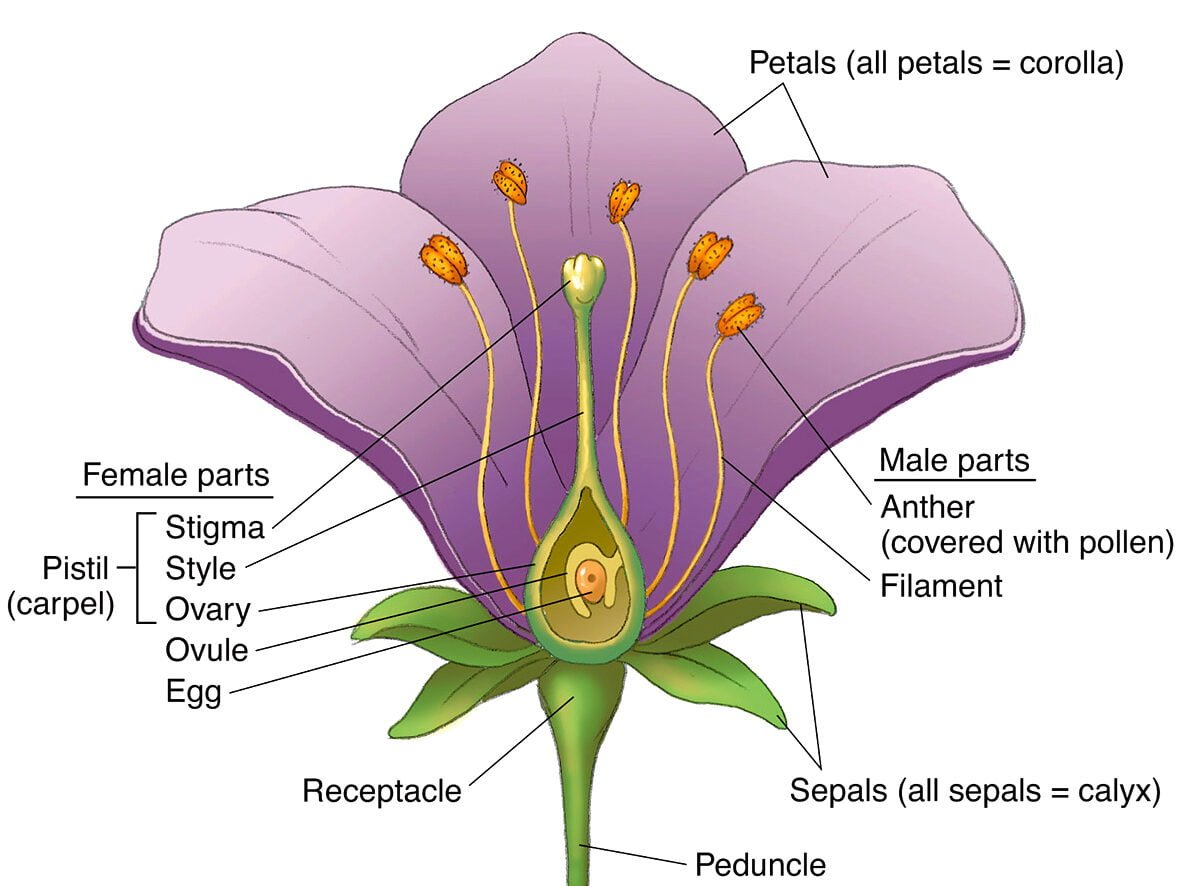
Electrical Energy

Definition:

Electrical energy is the work done by moving electric charges through a potential difference, converting electrical potential energy to other forms (heat, light, mechanical).

Energy Equations

\[ W = QV \]

\[ W = VIt \]

\[ W = I^2Rt \]

Where:
W = Energy (Joules)
Q = Charge (Coulombs)
V = Potential difference (Volts)
I = Current (Amperes)
t = Time (seconds)

Practical Units

Commercial Unit:

\[ 1 \, kWh = 3.6 \times 10^6 \, J \]

(1 kilowatt-hour = 1000 watts × 3600 seconds)

1 Joule = work done when 1A current flows through 1Ω for 1s
Electricity bills use kWh for energy measurement
1 calorie = 4.184 J (for heat energy calculations)

Electrical Power

Definition:

Electrical power is the rate at which electrical energy is transferred by an electric circuit, measured in Watts (Joules/second).

Power Equations

\[ P = \frac{W}{t} = VI \]

\[ P = I^2R \]

\[ P = \frac{V^2}{R} \]

Power Dissipation

In resistors: Power is dissipated as heat (Joule heating)
In motors: Converted to mechanical work + heat
In LEDs: Converted to light + heat
Maximum power transfer occurs when R_load = R_source

Power in Different Configurations

Circuit Element	Power Formula	Energy Conversion
Resistor	I²R or V²/R	Heat
Battery (discharging)	VI	Chemical → Electrical
Motor	VI – I²R	Electrical → Mechanical
LED	VI	Electrical → Light

Efficiency & Cost Calculations

Efficiency

\[ \eta = \frac{P_{out}}{P_{in}} \times 100\% \]

Examples:

Incandescent bulb: ~5% (95% heat)
LED bulb: ~30% (70% heat)
Electric motor: 70-95%
Power lines: ~98%

Energy Cost

\[ \text{Cost} = \frac{P(kW) \times t(h) \times \text{Rate}(Rs/kWh)}{1000} \]

Example Calculation:

200W fridge running 24/7 at ₹5/kWh:

\[ \frac{200 \times 24 \times 30 \times 5}{1000} = ₹720/month \]

Worked Example

Problem:

A 1.5kW electric kettle operates at 220V and takes 10 minutes to boil water. Calculate:

Current drawn
Resistance of heating element
Energy consumed in kWh
Cost to run 4 times/day at ₹6/kWh

Solution:

(1) Current drawn:

\[ I = \frac{P}{V} = \frac{1500}{220} \approx 6.82 \, A \]

(2) Resistance:

\[ R = \frac{V^2}{P} = \frac{220^2}{1500} \approx 32.27 \, Ω \]

(3) Energy per use:

\[ W = Pt = 1.5 \, kW \times \frac{10}{60} \, h = 0.25 \, kWh \]

(4) Daily cost:

\[ \text{Cost} = 4 \times 0.25 \times 6 = ₹6 \, \text{per day} \]

Practice Problems

Problem 1

A 100W bulb operates at 220V. Calculate its resistance and current drawn.

Problem 2

Calculate the monthly cost of running a 2kW AC for 8 hours daily at ₹8/kWh.

Problem 3

A motor draws 5A from 220V supply with 85% efficiency. Calculate output power and energy lost in 1 hour.

Problem 4

Compare the cost of running a 60W CFL and 15W LED for 10 years (6h/day) at ₹7/kWh.

Problem 5

A 1Ω resistor carries 5A current for 30 minutes. Calculate heat produced in joules and calories.

Key Takeaways

Essential Formulas

Energy: W = VIt = I²Rt = V²t/R
Power: P = VI = I²R = V²/R
1 kWh = 3.6×10⁶ J
1 cal = 4.184 J

Practical Considerations

Higher power devices need thicker wires
Energy efficiency saves money long-term
Heat dissipation must be managed in circuits
Power ratings indicate maximum safe operation

Next Topic: Electrical Resistivity & Conductivity

In the next section, we’ll explore the intrinsic properties of materials that determine their resistance to current flow.

Continue to Next Topic →