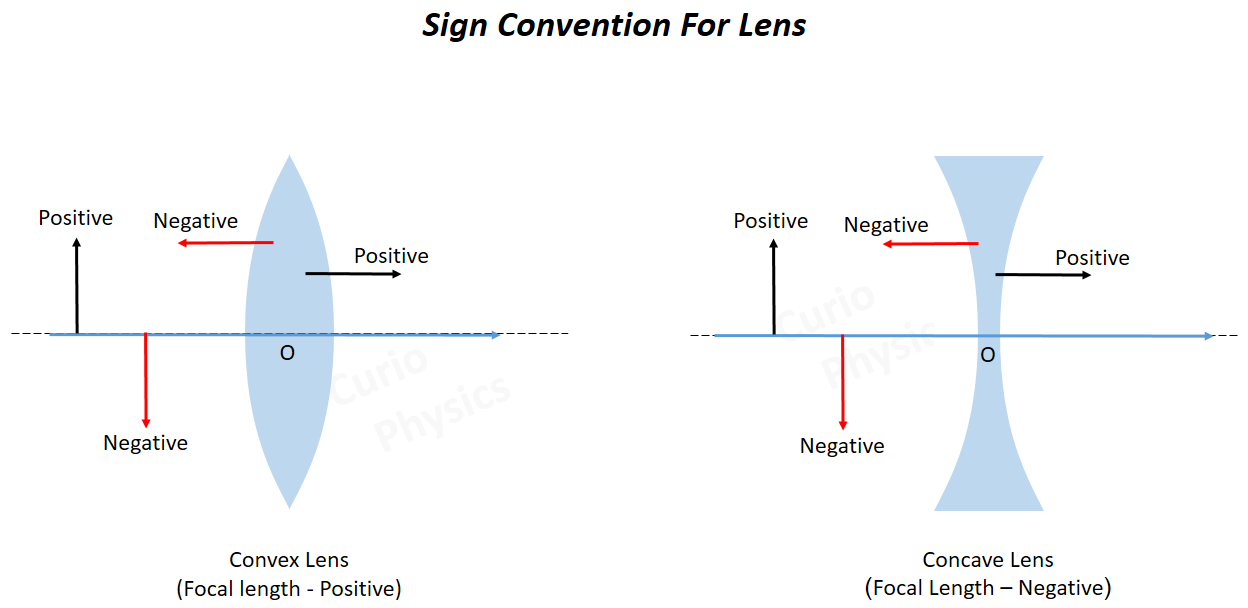
Sign Convention of Spherical Lenses
The sign convention for spherical lenses is essential for applying lens formulae correctly. It is a set of rules used to define the signs of distances (object distance, image distance) and focal length. The conventions for lenses are similar to those for mirrors, but there are some important differences due to the nature of light refraction through lenses.
Rules for Sign Convention of Spherical Lenses
- The optical center (O) of the lens is taken as the origin.
- All distances are measured from the optical center (O).
- Distances measured in the direction of the incident light (to the right) are taken as positive, and distances measured in the opposite direction (to the left) are taken as negative.
- Height measured upwards from the principal axis is considered positive, and height measured downwards is considered negative.
- The focal length of a convex lens is positive, while the focal length of a concave lens is negative.
 >
>Lens Formula
The lens formula, used to relate the object distance (u), image distance (v), and focal length (f), is given by:
Where:
- f = Focal length of the lens (positive for convex, negative for concave)
- v = Image distance (positive if on the opposite side of the object for convex lenses)
- u = Object distance (always negative for real objects)
Magnification Formula
Magnification (m) is the ratio of the height of the image to the height of the object. It is also related to the object and image distances:
Where:
- m = Magnification (positive for upright images, negative for inverted images)
- v = Image distance
- u = Object distance
- hi = Height of image
- ho = Height of object
Frequently Asked Questions (FAQs)
1. What is the sign convention for the object distance in lenses?
The object distance (u) is always negative in lenses if the object is real because it is placed on the left of the optical center.
2. How is the focal length of a concave lens signed?
The focal length (f) of a concave lens is always negative because the focus is on the same side as the object.
3. Why is the focal length of a convex lens positive?
The focal length of a convex lens is positive because it converges light, and the focus is on the opposite side of the object.
4. How do we determine the sign of image distance in lenses?
The image distance (v) is positive if the image is formed on the opposite side of the object (for real images) and negative if on the same side (for virtual images).
5. What is the lens formula, and when is it used?
The lens formula is 1/f = 1/v – 1/u, and it relates the object distance, image distance, and focal length. It is used to calculate the unknown parameter when two are known.
6. How is magnification signed in lenses?
Magnification (m) is positive for virtual and upright images and negative for real and inverted images.
7. Why are all distances measured from the optical center in lenses?
The optical center serves as the reference point, and all measurements are made relative to it to maintain consistency in the sign convention.
8. Can magnification be greater than 1?
Yes, magnification can be greater than 1 when the image is larger than the object, which happens when the object is placed between the focus and the optical center in convex lenses.
9. What happens if the object is placed at the focus of a convex lens?
If the object is placed at the focus of a convex lens, no image is formed as the refracted rays become parallel.
10. How is the height of an image related to the object in spherical lenses?
The height of the image is related to the object through magnification. If magnification is positive, the image is upright, and if negative, the image is inverted.