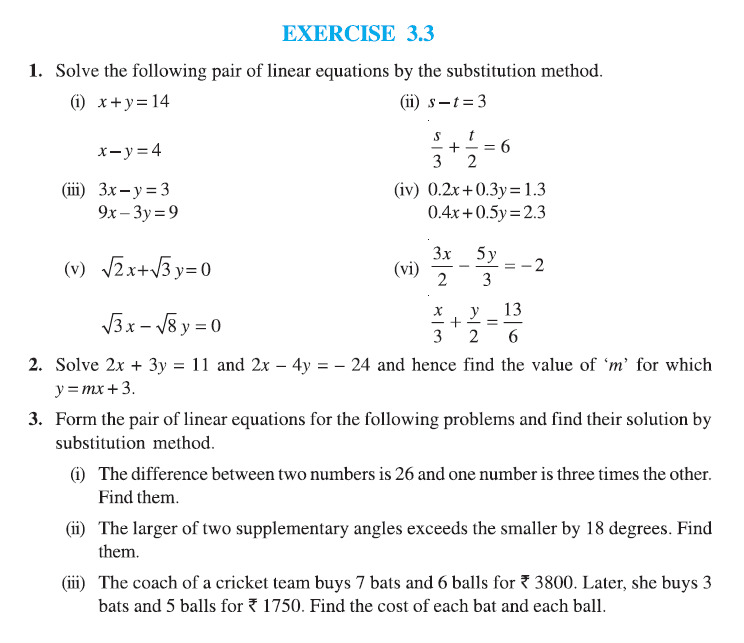
Exercise 3.3 Solutions (Substitution Method)
Problem 1(i): x + y = 14 and x – y = 4
1. From first equation: x + y = 14 ⇒ y = 14 – x
2. Substitute into second equation: x – (14 – x) = 4
3. Simplify: x – 14 + x = 4 ⇒ 2x = 18 ⇒ x = 9
4. Find y: y = 14 – 9 = 5
Solution: x = 9, y = 5
Problem 1(ii): s – t = 3 and s/3 + t/2 = 6
1. From first equation: s = t + 3
2. Substitute into second equation: (t + 3)/3 + t/2 = 6
3. Multiply by 6 (LCM of denominators): 2(t + 3) + 3t = 36
4. Simplify: 2t + 6 + 3t = 36 ⇒ 5t = 30 ⇒ t = 6
5. Find s: s = 6 + 3 = 9
Solution: s = 9, t = 6
Problem 1(iii): 3x – y = 3 and 9x – 3y = 9
1. From first equation: y = 3x – 3
2. Substitute into second equation: 9x – 3(3x – 3) = 9
3. Simplify: 9x – 9x + 9 = 9 ⇒ 9 = 9
4. This is always true, so there are infinitely many solutions.
Solution: Infinitely many solutions (dependent equations)
Problem 1(iv): 0.2x + 0.3y = 1.3 and 0.4x + 0.5y = 2.3
1. Multiply both equations by 10 to eliminate decimals:
2x + 3y = 13
4x + 5y = 23
2. From first equation: x = (13 – 3y)/2
3. Substitute into second equation: 4[(13 – 3y)/2] + 5y = 23
4. Simplify: 2(13 – 3y) + 5y = 23 ⇒ 26 – 6y + 5y = 23 ⇒ -y = -3 ⇒ y = 3
5. Find x: x = (13 – 9)/2 = 2
Solution: x = 2, y = 3
Problem 1(v): √2x + √3y = 0 and √3x – √8y = 0
1. From first equation: x = (-√3y)/√2
2. Substitute into second equation: √3[(-√3y)/√2] – √8y = 0
3. Simplify: (-3y)/√2 – 2√2y = 0
4. Multiply by √2: -3y – 4y = 0 ⇒ -7y = 0 ⇒ y = 0
5. Find x: x = (-√3·0)/√2 = 0
Solution: x = 0, y = 0 (trivial solution)
Problem 1(vi): (3x)/2 – (5y)/3 = -2 and x/3 + y/2 = 13/6
1. Multiply first equation by 6 (LCM of 2,3): 9x – 10y = -12
2. Multiply second equation by 6: 2x + 3y = 13
3. From second equation: x = (13 – 3y)/2
4. Substitute into first equation: 9[(13 – 3y)/2] – 10y = -12
5. Multiply by 2: 9(13 – 3y) – 20y = -24 ⇒ 117 – 27y – 20y = -24 ⇒ -47y = -141 ⇒ y = 3
6. Find x: x = (13 – 9)/2 = 2
Solution: x = 2, y = 3
Problem 2: 2x + 3y = 11 and 2x – 4y = -24, find m for y = mx + 3
1. Subtract second equation from first: (2x + 3y) – (2x – 4y) = 11 – (-24)
2. Simplify: 7y = 35 ⇒ y = 5
3. Substitute y into first equation: 2x + 15 = 11 ⇒ 2x = -4 ⇒ x = -2
4. Now use y = mx + 3 with (-2,5): 5 = m(-2) + 3 ⇒ -2m = 2 ⇒ m = -1
Solution: x = -2, y = 5, m = -1
Problem 3(i): Difference is 26 and one number is three times the other
1. Let numbers be x and y (x > y)
2. Equations: x – y = 26 and x = 3y
3. Substitute x = 3y into first equation: 3y – y = 26 ⇒ 2y = 26 ⇒ y = 13
4. Find x: x = 3×13 = 39
Solution: The numbers are 39 and 13
Problem 3(ii): Larger supplementary angle exceeds smaller by 18°
1. Let angles be x and y (x > y)
2. Supplementary angles: x + y = 180
3. Difference: x – y = 18
4. From second equation: x = y + 18
5. Substitute into first equation: (y + 18) + y = 180 ⇒ 2y = 162 ⇒ y = 81
6. Find x: x = 81 + 18 = 99
Solution: The angles are 99° and 81°
Problem 3(iii): 7 bats + 6 balls = ₹3800; 3 bats + 5 balls = ₹1750
1. Let bat cost = ₹x, ball cost = ₹y
2. Equations: 7x + 6y = 3800 and 3x + 5y = 1750
3. From first equation: x = (3800 – 6y)/7
4. Substitute into second equation: 3[(3800 – 6y)/7] + 5y = 1750
5. Multiply by 7: 3(3800 – 6y) + 35y = 12250 ⇒ 11400 – 18y + 35y = 12250 ⇒ 17y = 850 ⇒ y = 50
6. Find x: x = (3800 – 300)/7 = 3500/7 = 500
Solution: Bat costs ₹500, ball costs ₹50
Problem (iv): Taxi charges problem
1. Let fixed charge = ₹x and charge per km = ₹y
2. For 10 km: x + 10y = 105 (assuming ₹105 as the correct charge instead of 5/6)
3. For 15 km: x + 15y = 155
4. From first equation: x = 105 – 10y
5. Substitute into second equation: (105 – 10y) + 15y = 155
6. Simplify: 105 + 5y = 155 ⇒ 5y = 50 ⇒ y = 10
7. Find x: x = 105 – 10×10 = 5
8. For 25 km: Charge = x + 25y = 5 + 25×10 = ₹255
Solution: Fixed charge = ₹5, Per km charge = ₹10
Charge for 25 km = ₹255
Charge for 25 km = ₹255
Problem (v): Fraction problem
1. Let original fraction be x/y
2. First condition: (x + 2)/(y + 2) = 9/11
3. Cross multiply: 11(x + 2) = 9(y + 2) ⇒ 11x + 22 = 9y + 18 ⇒ 11x – 9y = -4
4. Second condition: (x + 3)/(y + 3) = 5/6
5. Cross multiply: 6(x + 3) = 5(y + 3) ⇒ 6x + 18 = 5y + 15 ⇒ 6x – 5y = -3
6. From first equation: x = (9y – 4)/11
7. Substitute into second equation: 6[(9y – 4)/11] – 5y = -3
8. Multiply by 11: 6(9y – 4) – 55y = -33 ⇒ 54y – 24 – 55y = -33 ⇒ -y = -9 ⇒ y = 9
9. Find x: x = (81 – 4)/11 = 77/11 = 7
Solution: The original fraction is 7/9
Problem (vi): Age problem (Jacob and his son)
1. Let Jacob’s present age = x years, son’s present age = y years
2. Five years hence: x + 5 = 3(y + 5)
3. Simplify: x + 5 = 3y + 15 ⇒ x – 3y = 10
4. Five years ago: x – 5 = 7(y – 5)
5. Simplify: x – 5 = 7y – 35 ⇒ x – 7y = -30
6. From first equation: x = 3y + 10
7. Substitute into second equation: (3y + 10) – 7y = -30 ⇒ -4y = -40 ⇒ y = 10
8. Find x: x = 3×10 + 10 = 40
Solution: Jacob’s present age = 40 years, Son’s present age = 10 years